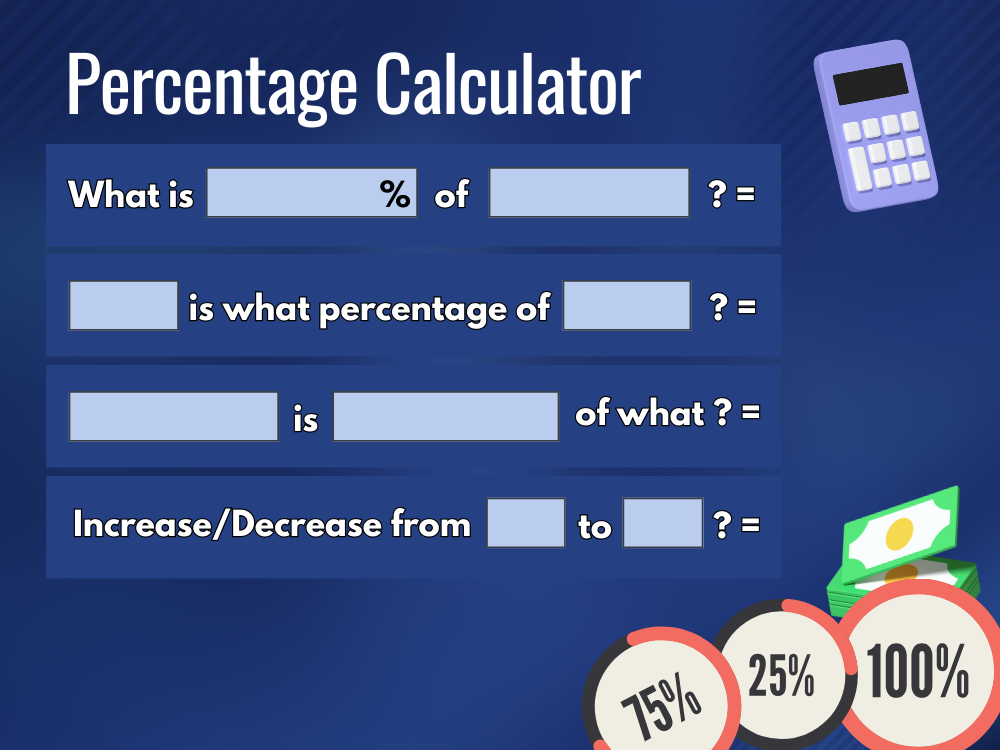
Percentage Calculator is a free online tool that can calculate percentages.
Percentage Calculator
You can type numbers into the calculator and it will show the percentage results immediately.
Percentage Calculator in Common Phrases
What is X% of Y?
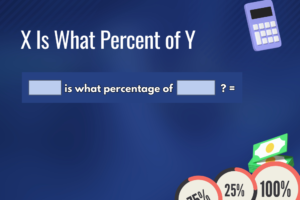
X Is What Percent of Y?
X is Y% of What?
Percentage Change Calculator
Calculating percentages can be useful in many areas of life, from figuring out sales tax to determining exam scores. Having a solid understanding of percentages can help with math classes, personal finances, work tasks, and more. This article will provide an in-depth look at percentages and how to calculate them accurately.
What is a Percentage?
A percentage represents a proportion of a whole. It expresses an amount as a fraction of 100. The word “percent” means “per 100” or “for every hundred”. So a percentage is just another way to express a fraction with a denominator of 100.
For example, if there are 500 students in a school and 50 of them play soccer, the percentage of students who play soccer is:
(50 students who play soccer/500 total students) x 100 = 10%
So 10% of the students play soccer.
Some key notes about percentages:
- They can take values from 0% to 100%. Higher percentages mean larger portions.
- 0% means none. 100% means all.
- Percentages can be greater than 100% or less than 0%, but these cases are less common.
Converting Percentages, Fractions, and Decimals
Percentages, fractions, and decimals are related concepts. It’s helpful to understand how to convert between them:
Percentage to Fraction
To convert a percentage to a fraction, put the percentage over 100 and simplify:
For example,
50% is 50/100 = 1/2.
75% is 75/100 = 3/4.
Fraction to Percentage
To go from a fraction to a percentage, divide the numerator by the denominator and multiply by 100:
For example,
2/5 = (2/5) * 100 = 40%
3/10 = (3/10) * 100 = 30%
Percentage to Decimal
To convert a percentage to a decimal, divide it by 100:
For example,
25% = 25/100 = 0.25
50% = 50/100 = 0.50
60% = 60/100 = 0.60
Decimal to Percentage
To convert a decimal to a percentage, multiply it by 100:
For example,
0.30 = 0.30 * 100 = 30%
0.45 = 0.45 * 100 = 45%
0.80 = 0.80 * 100 = 80%
Practice converting between percentages, decimals, and fractions to get comfortable with these relationships.
Calculating Percentages
There are a few basic methods for calculating percentages.
Method 1: Percentage of a Number
To calculate a percentage of a number:
- Convert the percentage to a decimal
- Multiply the decimal by the number
For example, to find 25% of 20:
- 25% = 0.25 (convert to decimal)
- 0.25 * 20 = 5
So 25% of 20 is 5.
This method works for finding any percentage of any number. Some more examples:
- 10% of 50 = 0.10 * 50 = 5
- 2% of 300 = 0.02 * 300 = 6
- 110% of 15 = 1.1 * 15 = 16.5
Method 2: Part-Whole Method
If you know the percentage and the total or whole, you can use the part-whole method:
Part = Percentage x Whole / 100
For example, if you scored 18 out of 25 points on a test, what percentage did you get correct?
- The part is your score: 18
- The whole is the total points: 25
- The percentage is unknown
Plugging into the formula:
Part = Percentage x Whole / 100
18 = Percentage x 25 / 100
0.72 = Percentage
You got 72% of the test correct.
This method is useful when you know the part and the whole, and need to calculate the percentage.
Method 3: Finding the Whole
You can also use the part-whole method in reverse, to find the whole if you know the part and percentage:
Whole = Part x 100 / Percentage
For example, you got 8 out of some total points on an exam, and your score was 80%. How many total points was the exam worth?
- The part is the points you got: 8
- The percentage is 80%
- The whole is unknown
Plugging into the formula:
Whole = Part x 100 / Percentage
Whole = 8 x 100 / 80 Whole = 10
If you got 8 out of 80%, the whole exam must have been 10 points.
This method lets you calculate the total whole from a part and percentage.
Applications of Percentages
Some common real-world uses of percentages include:
- Sales Tax – Sales tax is added as a percentage of the original price. For example, with a sales tax of 8%, you would calculate 8% of the pre-tax total.
- Tips – Tips at restaurants are often calculated as a percentage of the bill. A common tip is 15-20%.
- Commissions – Commission workers like salespeople often earn a percent of each sale. For example, earning 5% commission on $1000 in sales would be 0.05 * $1000 = $50.
- Interest Rates – Interest rates on loans or savings accounts are expressed as annual percentage rates. This interest is calculated as a percent of the principal amount.
- Exam Scores – Exam scores are frequently calculated as the number of points earned divided by total points, and then converted to a percentage.
- Percentiles – When data is ranked, percentiles express an observation’s value as a percent of the whole set. For example, the 80th percentile means the value is greater than 80% of the data.
Mastering percentage calculations is very helpful for working with statistics, business applications, and more!
Percentage Increase and Decrease
Two other important percentage operations are increases and decreases. These calculate the change from one value to another as a percentage.
Percentage Increase Formula
Percentage Increase = (New Value – Original Value) / Original Value x 100
This formula finds the amount of increase as a percentage of the original amount.
For example, if the price of an item increased from $100 to $150:
Percentage Increase = ($150 – $100) / $100 x 100 = 0.5 x 100 = 50%
The price increased by 50%.
Percentage Decrease Formula
Percentage Decrease = (Original Value – New Value) / Original Value x 100
This formula determines the percentage decrease from the original amount to the new amount.
For example, if the price of an item decreased from $80 to $60:
Percentage Decrease = ($80 – $60) / $80 x 100 = 0.25 x 100 = 25%
The price decreased by 25%.
Calculating percentage changes is useful for comparing business metrics, investment returns, and more.
Percentage Error
Another important concept is percentage error, which measures how far an experimental measurement deviates from the true value:
Percentage Error = |Measured Value – True Value| / True Value x 100
The absolute value accounts for the measured value being either too high or too low.
For example, if you measured a distance as 12.1 inches, but the actual distance was 11.8 inches:
Percentage Error = |12.1 – 11.8| / 11.8 x 100 = 0.3 / 11.8 x 100 = 2.5%
This percentage error of 2.5% shows how close the measurement was to the true distance.
Scientists commonly use percentage error to evaluate the accuracy of experimental results compared to true values. The smaller the percentage error, the more accurate the measurement.
Conclusion
In summary, a percentage is a ratio expressed as a fraction out of 100. There are straightforward methods to calculate percentages of numbers, increases and decreases, part-whole relationships, and percentage errors. Converting between percentages, decimals fractions facilitates these calculations. Online calculators can assist with tricky percentage problems. Getting comfortable working with percentages takes practice across diverse types of problems. Understanding percentages is essential for business, math, science, and many real-world situations.